|

Scott,
I would like to know what the
formula is for an amortized loan. How exactly do they calculate each
payment? I suspect that all the interest for the loan term (i.e., 30
years, 15 years, etc.) is loaded up front in some way, but I would
like to know exactly HOW? I want to teach my teenagers about this. I
think it's very important for everyone to know.
If everyone understood these
things that affect us all, there would be fewer people getting into
financial trouble.
Thank you.
--Maria
Maria,
 Thanks for writing! That's a great
question and one I will truly enjoy answering! My background is in
Engineering and math and I do love math. It's a good thing too,
because doing the math has helped me make the right buying decisions
and avoid being tricked countless times. Thanks for writing! That's a great
question and one I will truly enjoy answering! My background is in
Engineering and math and I do love math. It's a good thing too,
because doing the math has helped me make the right buying decisions
and avoid being tricked countless times.
However, I must warn that the
explanation of the formula to calculate loans is quite involved so
be ready to get an Algebra refresher.
First thing to address is your
suspicion that loans are "loaded up front." You can think
of it this way but it's better think in terms of periodic (monthly),
discrete transactions. The way all loans work is quite simple.
At some periodic time, usually
monthly, the amount owed is multiplied by the periodic interest
rate. That amount is then added to the original amount owed and any
payments are subtracted. That's it. The mathematical formula is:
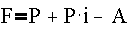
Equation #1
Where:
F = Future value of an annuity (unpaid balance)
P = Principal value
i = Periodic interest (APR divided by 12)
n = Number of periods (months for most loans)
A = Periodic payment (monthly payment for most loans)
For example:
Say you have a $10,000 loan at 12% APR. At the end of the first
month the bank is going to calculate the interest by multiplying
$10,000 by the periodic rate (monthly rate). The monthly rate is 1%
because 12x1%=12%APR. So the interest charge is 1% of $10,000 or
$100. That amount is added to the balance so the new balance is
$10,100 or:
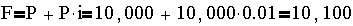
From this amount the bank will
subtract your payments (A). It is apparent that you must pay more
than $100 or you'll be in a situation where the balance will never
decrease. If you pay $100 then that would be subtracted from the new
balance and you're back to owing $10,000. At that rate the loan will
never be paid off.
To pay off the loan in a specific
number of periods (months) you must make a certain payment that's
greater than the periodic interest charge.
The formula to find the monthly
payment (A) to pay off a loan in n periods is:
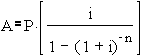
Equation #2
The formula to find the future
balance (unpaid balance) of a loan is:
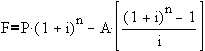
Equation #3
Notice that if F=0 in equation #3,
and we solve for A, we get equation #2.
Granted, equations 2 and 3 don't look
as simple as equation 1, but keep in mind that they are based on that
simple equation 1.
Let's use these formulas in the first
example. Say you want to pay off that $10,000, 12% APR loan in
exactly 5 years. What payment do you have to make to do this?
Let's list the variables:
P = $10,000
i = 1% (APR divided by 12)
n = 60 months (5 years)
A = Periodic
payment (monthly payment). This is what we're looking to find.
To find the monthly payment use
equation #2 as follows:
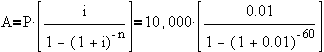
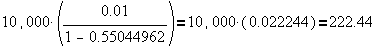
The monthly payment for this loan is
$222.44. Now let's say you want to find out how much you owe on the
loan after making 15 monthly payment.
Let's list the variables:
P = $10,000
i = 1% (APR divided by 12)
n = 15 months
A = $222.44
Using equation #3 as follows:
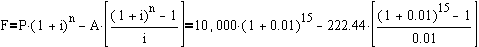

After 15 payments of $222.44 the
unpaid balance remaining is $8,029.03.
Conclusion:
This article discussed
how to use the formulas associated with finding the payment and
amortization of annuities (loans). I'll save the derivation of these
functions for another article.
Hope that helps!
Talk to you later.
Regards,
Scott
--End--
| 